
Victory Process 2D – A Valuable Alternative To SUPREM-based Simulators
SUPREM-IV – Stanford University PRocEss Modeling Program was first released 30 years ago. Since then its descendants - Athena from Silvaco and TSUPREM-4TM from TMA/Avant/Synopsys - have been 2D process simulation “work-horses” in semiconductor industry world-wide. Despite the fact that tremendous progress in the industry indeed requires the transition to 3D TCAD there are still many technologies and applications where a 2D simulation is the most practical approach. At the same time, we have to recognize that the SUPREM-based simulators cannot keep up with the progress because they lack many fundamental capabilities required for simulation of modern processes. Victory Process (VP) has been developed by Silvaco to address these challenging requirements. Though ultimate purpose of VP is accurate simulation of complex 3D process it still can be used as a valuable alternative to or even advantageous substitution for SUPREM based simulators. The 2D-mode of Victory Process (VP2D) differs from the full 3D mode only by setting a 2D simulation domain when simulation starts. This means that the same syntax, models, algorithms, layout and the whole process flow can be used in both 2D and 3D. This guarantees smooth transition from 2D to 3D process simulation.
Simulation of Device Degradation Due to Bias Temperature Stress
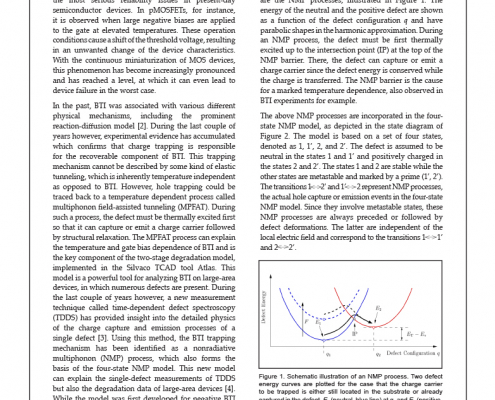
Introduction Bias Temperature Instability (BTI) [1] ranks among the most serious reliability issues in present-day semiconductor devices. In pMOSFETs, for instance, it is observed when large negative biases are applied to the gate at elevated temperatures. These operation conditions cause a shift of the threshold voltage, resulting in an unwanted change of the device characteristics. With the continuous miniaturization of MOS devices, this phenomenon has become increasingly pronounced and has reached a level, at which it can even lead to device failure in the worst case.

Using Victory Process to Create Realistic Structures for Capacitance Extraction in Clever
Silvaco offers many alternatives for creating simple 3D structures, the optimum choice depending on what the user needs to simulate. Many of the choices for creating simple 3D structures are for user convenience, so that just a single tool can both create the simple 3D structure and simulate the required physics. This gives the user an enhanced feeling of a tightly integrated product.

Hints, Tips and Solutions – How to best visualize vectors and field lines in TonyPlot
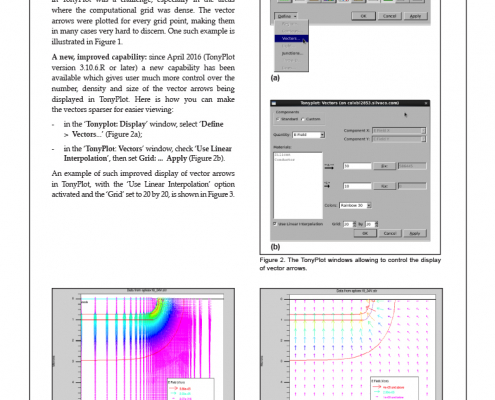
Traditionally, visualizing vectors by means of arrows in TonyPlot was a challenge, especially in the areas where the computational grid was dense. The vector arrows were plotted for every grid point, making them in many cases very hard to discern. One such example is illustrated in Figure 1.
Hints, Tips and Solutions – Scattering mechanisms contributing to reduced channel mobility in 4H-SiC MOSFETs
In a MOSFET structure, silicon carbide, 4H-SiC in particular, is known to exhibit lower channel mobility than Si, mainly due to Coulomb scattering at trapped charge at the SiO2/4H-SiC interface, where a high interface trap density exists. Atlas provides an alternative inversion layer mobility model specifically intended for 4H-SiC. The model enabled by specifying the ALTCVT.N parameter for electrons or the ALTCVT.P parameter for holes on the MOBILITY statement takes into account four scattering mechanisms. These comprise the ionized impurity scattering in the bulk semiconductor, the surface roughness scattering, the acoustic surface phonon scattering, and the Coulomb scattering at trapped charge at the SiO2/4H-SiC interface. Using Matthiessen’s rule, the ALTCVT.N and ALTCVT.P model combines four mobility components related to their respective carrier scattering to form the total inversion layer mobility in 4H-SiC.

Soft Error
A soft error in the context of this article, can be defined as an unintended change in electrical state of a device or circuit, that has an origin, external to the system’s designed inputs and outputs. A “soft” error is one which causes no direct permanent damage to the systems components, such that the unintended system behavior can be corrected with some form of “re-set”.
Hints, Tips and Solutions – Types of 3D Delaunay Shape Refinement
Q: What Types of 3D Delaunay Shape Refinement can be used in Victory Process?
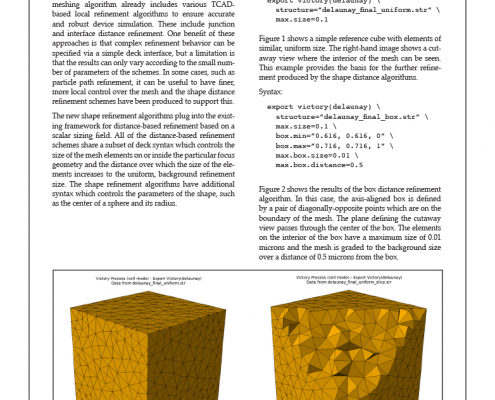
A. The Victory Process cell mode Delaunay 3D device meshing algorithm already includes various TCAD-based local refinement algorithms to ensure accurate and robust device simulation. These include junction and interface distance refinement. One benefit of these approaches is that complex refinement behavior can be specified via a simple deck interface, but a limitation is that the results can only vary according to the small number of parameters of the schemes. In some cases, such as particle path refinement, it can be useful to have finer, more local control over the mesh and the shape distance refinement schemes have been produced to support this.
Blue LED Simulation
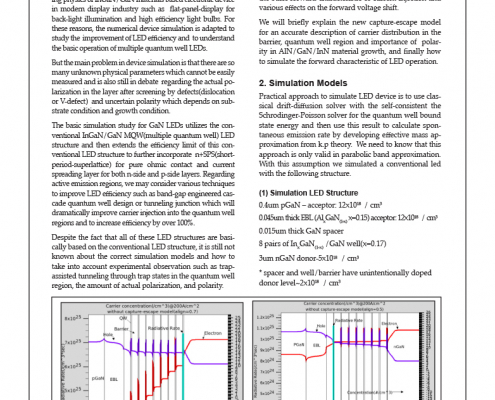
It is very important to understand the operation and underlying physics of InGaN/GaN materials based electronic device in modern display industry such as flat-panel-display for back-light illumination and high efficiency light bulbs. For these reasons, the numerical device simulation is adapted to study the improvement of LED efficiency and to understand the basic operation of multiple quantum well LEDs.
Unified Victory Conformal Export for 2D Process Mode
Victory Process can operate in either 1, 2 or 3-dimensions and can produce one of two different geometric representations: cell mode and process mode. Cell mode structures are generally composed of large, flat geometric parts but process mode structures may be smoothly varying and only very locally flat. Device meshes of cell mode structures resolve the input shape precisely but this is undesirable for the case of process mode structures as it would result in very finely sampled meshes which would be unsuitable for device simulation.
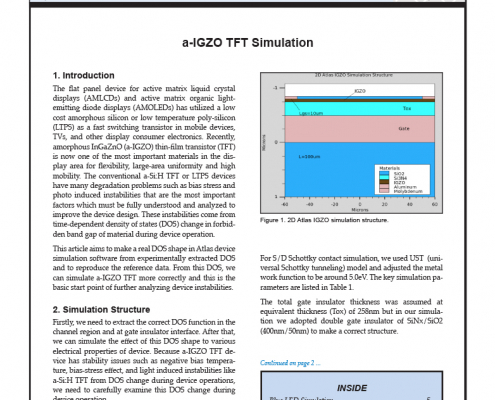
a-IGZO TFT Simulation
The flat panel device for active matrix liquid crystal displays (AMLCDs) and active matrix organic light-emitting diode displays (AMOLEDs) has utilized a low cost amorphous silicon or low temperature poly-silicon (LTPS) as a fast switching transistor in mobile devices,TVs, and other display consumer electronics. Recently, amorphous InGaZnO (a-IGZO) thin-film transistor (TFT) is now one of the most important materials in the display area for flexibility, large-area uniformity and high mobility. The conventional a-Si:H TFT or LTPS devices have many degradation problems such as bias stress and photo induced instabilities that are the most important factors which must be fully understood and analyzed to improve the device design. These instabilities come from time-dependent density of states (DOS) change in forbidden band gap of material during device operation.
